Members of the electromagnetic field mapping group in Zeuthen in June 2018
Members of the electromagnetic field mapping group in the ER-C in February 2017

Group members

Name Role
Rafal E. Dunin-Borkowski Professor; Director of PGI-5
András Kovács Permanent scientific staff
Yoshie Murooka Scientific staff
Amir H. Tavabi Scientific staff
Thibaud Denneulin Scientific staff
Qianqian Lan Scientific staff
Janghyun Jo Scientific staff
Fengshan Zheng Scientific staff
Benjamin Zingsem Scientific staff
Peng-Han Lu PhD student
Teresa Wessels PhD student
Tanvi Bhatnagar PhD student with JCNS?
Giulio Pozzi Visiting Professor
Vincenzo Grillo Visiting Scientist

Former group members

Name Role Period
Dongsheng Song Scientific staff 2018 - 2020
Elisabeth Josten Scientific staff
Vadim Migunov Scientific staff 2014 - 2020
Jan Caron Scientific staff
Patrick Diehle PhD student
Trevor Almeida Visiting Scientist

Research fields

Electric field mapping

Projected electrostatic potential and charge density mapping

(a) Cumulative charge profile measured along the electrically biased atom probe needle for an applied bias of 5 V, with the counter-electrode at distance of approximately 1 µm. The black line shows the result of model-independent approach using contour integration. The red line shows the result of model-based fitting using a line of constant charge density. (b) Reconstructed electron-optical phase after subtracting contributions from the mean inner potential and the magnetic field of the specimen. The edge of the needle is outlined with a black dashed line. The red and cyan boxes show integration contours. Regions marked in colour in (a) and (b) correspond to different charge densities identified from the black line profile in (a). (Only half of the tip is marked in colour in (b) to allow the phase distribution in the other half of the tip to be seen). (c) Charge profile measured across the needle using the cyan integration contour marked in (b).

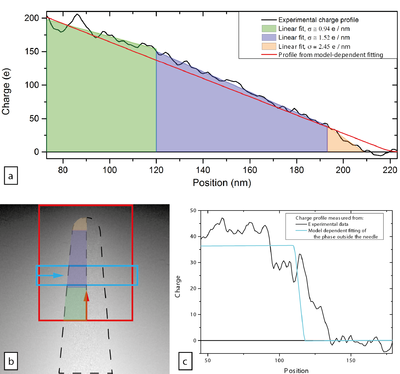
The distribution of electric field around a needle shaped sample is crucial information for design of novel field emitting devices and atom probe tomography (APT).

We have used off-axis electron holography combined with in situ electrical biasing to measure distribution of projected electrostatic potential which is encoded in the electron phase shift. [1] The analyses of the data can be performed using both model-dependent and model-independent approach. The first one utilises fitting of projected electrostatic potential to analytical model for line of charges which describes potential distribution around charged rotational ellipsoid. [2] The model-independent approach relies on calculation of projected charge density from the phase maps by using either 2D Laplacian operator [3] or by loop integration[4]. Both approaches can be combined: model-independent one can be used to refine the model and with assumption of symmetry, refined model can be used for calculations of 3D potential distribution.

Performing full three-dimensional reconstruction of potential or charge density would take this work forward.

Further reading: [5]

Further contact: Vadim Migunov, Fengshan Zheng

Three dimensional mapping of electrostatic potential, electric field and charge density

  • Analytic model-dependent approach[6]
  • Model-independent approach[7]
  • Model-based iterative reconstruction[8]

Further contact: Fengshan Zheng, Vadim Migunov

Magnetic field mapping

Magnetic vector field tomography

Shaping electron beams

In situ electron microscopy

Methods, instrumentation and software

Methods

Instrumentation

Software

Data analyses

Pages

References

  1. ↑ http://dx.doi.org/10.1063/1.4916609 Migunov, V., London, A., Farle, M. & Dunin-Borkowski, R. E. Model-independent measurement of the charge density distribution along an Fe atom probe needle using off-axis electron holography without mean inner potential effects. Journal of Applied Physics 117, 134301 (2015).
  2. ↑ http://doi.org/10.1016/0304-3991(92)90039-M Matteucci, G., Missiroli, G. F., Muccini, M. & Pozzi, G. Electron holography in the study of the electrostatic fields: the case of charged microtips. Ultramicroscopy 45, 77–83 (1992).
  3. ↑ http://dx.doi.org/10.1063/1.3598468 Beleggia, M., Kasama, T., Dunin-Borkowski, R. E., Hofmann, S. & Pozzi, G. Direct measurement of the charge distribution along a biased carbon nanotube bundle using electron holography. Applied Physics Letters 98, 243101 (2011).
  4. ↑ http://dx.doi.org/10.1103/PhysRevLett.111.025501 Gatel, C., Lubk, A., Pozzi, G., Snoeck, E. & Hÿtch, M. Counting Elementary Charges on Nanoparticles by Electron Holography. Phys. Rev. Lett. 111, 25501 (2013).
  5. ↑ http://dx.doi.org/10.1063/1.4916609 Migunov, V., London, A., Farle, M. & Dunin-Borkowski, R. E. Model-independent measurement of the charge density distribution along an Fe atom probe needle using off-axis electron holography without mean inner potential effects. Journal of Applied Physics 117, 134301 (2015).
  6. ↑ http://dx.doi.org/10.1063/1.4916609 Migunov, V., London, A., Farle, M. & Dunin-Borkowski, R. E. Model-independent measurement of the charge density distribution along an Fe atom probe needle using off-axis electron holography without mean inner potential effects. Journal of Applied Physics 117, 134301 (2015).
  7. ↑ http://dx.doi.org/10.1063/1.3598468 Beleggia, M., Kasama, T., Dunin-Borkowski, R. E., Hofmann, S. & Pozzi, G. Direct measurement of the charge distribution along a biased carbon nanotube bundle using electron holography. Applied Physics Letters 98, 243101 (2011).
  8. ↑ https://www.sciencedirect.com/science/article/pii/S0368204818302032 Zheng, F., Caron, J., Migunov, V., Beleggia, M., Pozzi, G. & Dunin-Borkowski, R. E. Measurement of charge density in nanoscale materials using off-axis electron holography. Journal of Electron Spectroscopy and Related Phenomena, in press.
Retrieved from "http://iffwiki.fz-juelich.de/index.php?title=PGI5:_Electromagnetic_field_mapping_working_group&oldid=5772"
Loading...
Imprint